-40%
Rare Torricelli founder o Hydrodynamics antique Theorem Demonstration Apparatus
$ 132
- Description
- Size Guide
Description
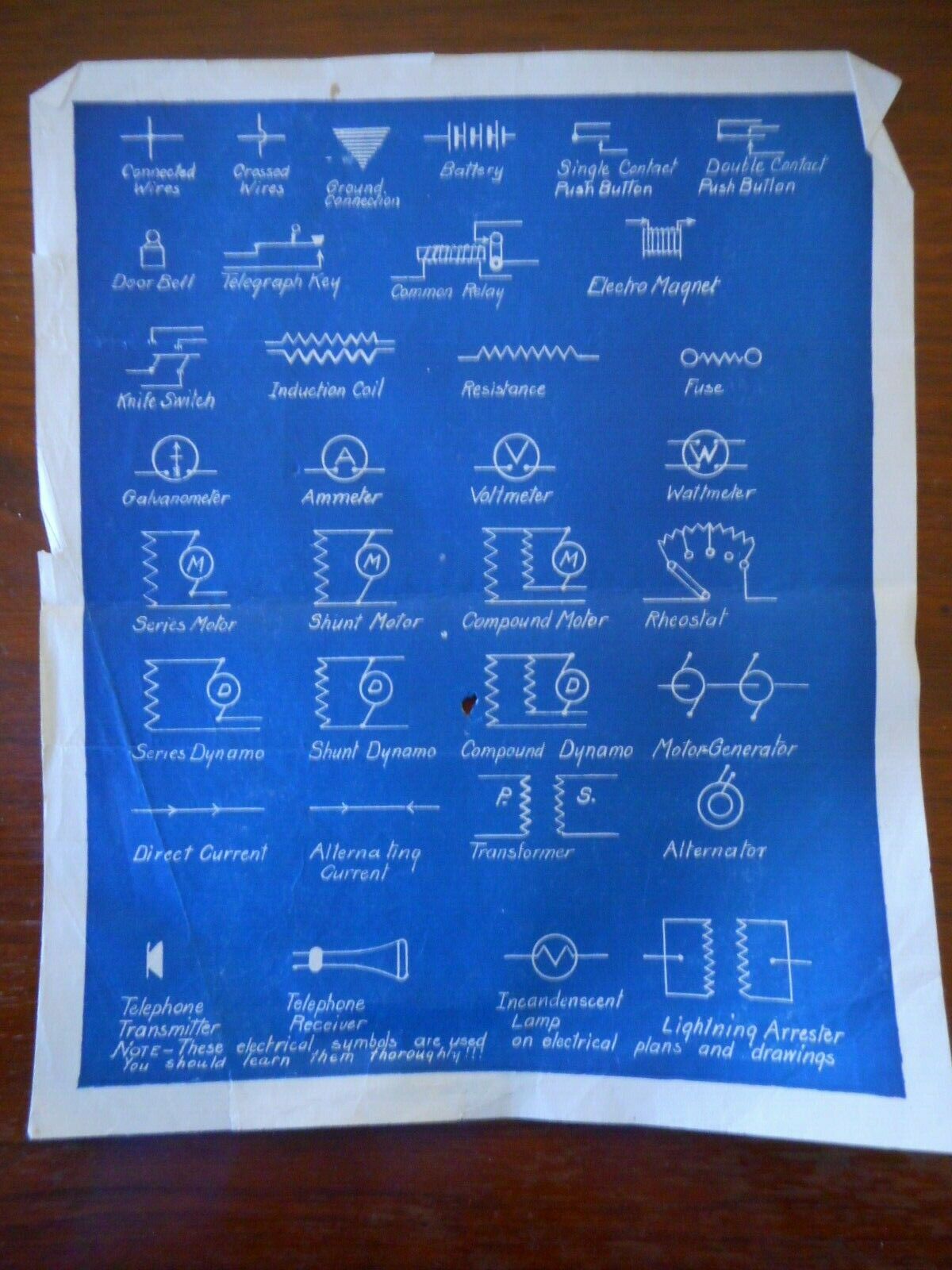
Rare Torricelli, founder of Hydrodynamics, antique Demonstration Apparatus to demonstrate the Torricelli TheoremTorricelli’s Theorem
Torricelli had interests in various aspects of physics and mathematics and torricelli’s theorem was one of his biggest achievements. The law explains the relation between fluid leaving a hole and the liquid’s height in that container.
The relationship can be summarized in the following manner. If you have a container filled with fluid with small holes at the bottom of the container, the fluid leaves through the hole with velocity same as it would experience if dropped from the same height to the hole level. If the liquid is dropped from a height h, it would have a velocity v and this v is the same velocity at which the liquid leaves the hole when the height of fluid h is same as liquid dropped in the container.
We also assume that it is an ideal fluid which means that the liquid is in compressible, non-viscous and laminar flow. These factors cannot be neglected since same rules cannot be applied to non-fluids as their viscosity and flow may not be same across the liquid itself. The apparatus has five valves to demonstrate the Torricelli Theorem.
Evangelista Torricelli, born: 15 October 1608 in Faenza, Romagna, died 25 October 1647 in Florence, Tuscany, Italy
Evangelista Torricelli's parents were Gaspare Torricelli and Caterina Angetti. It was a fairly poor family with Gaspare being a textile worker. Evangelista was the eldest of his parents three children, having two younger brothers at least one of whom went on to work with cloth. It is greatly to his parents' credit that they saw that their eldest son had remarkable talents and, lacking the resources to provide an education for him themselves, they sent him to his uncle who was a Camaldolese monk. Brother Jacopo saw that Evangelista was given a sound education until he was old enough to enter a Jesuit school.
Torricelli entered a Jesuit College in 1624 and studied mathematics and philosophy there until 1626. It is not entirely clear at which College he studied, with most historians believing that he attended the Jesuit College in Faenza, while some believe that he entered the Collegio Romano in Rome. What is undoubtedly the case is that after study at the Jesuit College he was then in Rome. Certain facts are clear, namely that Torricelli's father died in or before 1626 and that his mother moved to Rome for she was certainly living there in 1641 at the time of her death. Torricelli's two brothers also moved to Rome and again we know for certain that they were living there in 1647. The most likely events seem to be that after Gaspare Torricelli died, Caterina and her two younger sons moved to Rome to be with Evangelista who was either already living there or about to move to that city.
At the Jesuit College Torricelli showed that he had outstanding talents and his uncle, Brother Jacopo, arranged for him to study with Benedetto Castelli. Castelli, who like Jacopo was a Camaldolese monk, taught at the University of Sapienza in Rome. Sapienza was the name of the building which the University of Rome occupied at this time and it gave its name to the University. There is no evidence that Torricelli was actually enrolled at the university, and it is almost certain that he was simply being taught by Castelli as a private arrangement. As well as being taught mathematics, mechanics, hydraulics, and astronomy by Castelli, Torricelli became his secretary and held this post from 1626 to 1632. It was an arrangement which meant that he worked for Castelli in exchange for the tuition he received. Much later he took over Castelli's teaching when he was absent from Rome.
There does still exist a letter which Torricelli wrote to Galileo on 11 September 1632 and it gives us some very useful information about Torricelli's scientific progress. Galileo had written to Castelli but, since Castelli was away from Rome at the time, his secretary Torricelli wrote to Galileo to explain this fact. Torricelli was an ambitious young man and he greatly admired Galileo, so he took the opportunity to inform Galileo of his own mathematical work. Torricelli began by Galileo Galileo that he was a professional mathematician and that he had studied the classical texts of Apollonius, Archimedes and Theodosius. He had also read almost everything that the contemporary mathematicians Brahe, Kepler and Longomontanus had written and, he told Galileo, he was convinced by the theory of Copernicus that the Earth revolved round the sun. Moreover, he had carefully studied Dialogue Concerning the Two Chief Systems of the World - Ptolemaic and Copernican which Galileo had published about six months before Torricelli wrote his letter.
It was clear from his letter that Torricelli was fascinated by astronomy and was a strong supporter of Galileo. However the Inquisition banned the sale of the Dialogue and ordered Galileo to appear in Rome before them. After Galileo's trial in 1633, Torricelli realised that he would be on dangerous ground were he to continue with his interests in the Copernican theory so he deliberately shifted his attention onto mathematical areas which seemed less controversial. During the next nine years he served as secretary to Giovanni Ciampoli, a friend of Galileo, and possibly a number of other professors. We do not know where Torricelli lived during this period but, as Ciampoli served as governor of a number of cities in Umbria and the Marches, it is likely that he lived for periods in Montalto, Norcia, San Severino and Fabriano.
By 1641 Torricelli had completed much of the work which he was to publish in three parts as Opera geometrica Ⓣ in 1644. We shall give more details of this work later in this biography, but for the moment we are interested in the second of the three parts De motu gravium Ⓣ. This basically carried on developing Galileo's study of the parabolic motion of projectiles which had appeared in Discourses and mathematical demonstrations concerning the two new sciences published in 1638. Torricelli was certainly in Rome in early 1641 when he asked Castelli for his opinion on De motu gravium. Castelli was so impressed that he wrote to Galileo himself, at this time living in his home in Arcetri near Florence, watched over by officers from the Inquisition. In April 1641 Castelli travelled from Rome to Venice and, on the way, stopped in Arcetri to give Galileo a copy of Torricelli's manuscript and suggest that he employed him as an assistant.
Torricelli remained in Rome while Castelli was on his travels and gave his lectures in his place. Although Galileo was keen to have Torricelli's assistance there was a delay before this could happen. On the one hand Castelli did not return to Rome for some time, while the death of Torricelli's mother further delayed his departure. On 10 October 1641 Torricelli arrived at Galileo's house in Arcetri. He lived there with Galileo and also with Viviani who was already assisting Galileo. He only had a few months with Galileo, however, before that famous scientist died in January 1642. Delaying his return to Rome for a while after Galileo died, Torricelli was appointed to succeed Galileo as the court mathematician to Grand Duke Ferdinando II of Tuscany. He did not receive the title of Court Philosopher to the Grand Duke which Galileo had also held. He held this post until his death living in the ducal palace in Florence.
In looking at Torricelli's achievements we should first put his mathematical work into context. Another pupil of Castelli, Bonaventura Cavalieri, held the chair of mathematics at Bologna. Cavalieri presented his theory of indivisibles in Geometria indivisibilis continuorum nova published in 1635. The method was a development of Archimedes' method of exhaustion incorporating Kepler's theory of infinitesimally small geometric quantities. This theory allowed Cavalieri to find, in a simple and rapid way, the area and volume of various geometric figures. Torricelli studied the methods being proposed by Cavalieri and at first was suspicious of them. However, he soon became convinced that these powerful methods were correct and began to develop them further himself. In fact he used a combination of the new and old methods, using the method of indivisibles to discover his results, but often giving a classical geometrical proof of them. He gave this not because he doubted the correctness of the method of indivisibles, rather because he wanted to give a proof:-
... according to the usual method of the ancient geometers ...
so that readers not familiar with the new methods would still be convinced of the correctness of his results.
By 1641 he had proved a number of impressive results using the methods which he would publish three years later. He examined the three dimensional figures obtained by rotating a regular polygon about an axis of symmetry. Torricelli also computed the area and centre of gravity of the cycloid. His most remarkable results, however, resulted from his extension of Cavalieri's method of indivisibles to cover curved indivisibles. With these tools he was able to show that rotating the unlimited area of a rectangular hyperbola between the y-axis and a fixed point on the curve, resulted in a finite volume when rotated round the y-axis. Notice that we have stated this result in the modern notation of coordinate geometry which was totally unavailable to Torricelli. This last result, described in [1] as:-
... a gem of the mathematical literature of the time ...
is considered in detail in [23] where it is noted that, immediately after its publication in 1644, the result aroused great interest and admiration because it went totally against the intuition of mathematicians of the period.
We mentioned Torricelli's results on the cycloid and these resulted in a dispute between him and Roberval. The article [19] discusses:-
... a letter dated October 1643, by which Torricelli gets in touch with Roberval and reports to him about his views and results on the centre of gravity of the parabola, the semigeneral parabolas, the surface of the cycloid and its history, the solid of revolution generated by a conic and the hyperbolic acute solid.
We should also note another fine contribution made by Torricelli was in solving a problem due to Fermat when he determined the point in the plane of a triangle so that the sum of its distances from the vertices is a minimum (known as the isogonic centre of the triangle). This contribution, described in detail in [20], is summarised in that paper as follows:-
Around 1640, Torricelli devised a geometrical solution to a problem, allegedly first formulated in the early 1600s by Fermat: 'given three points in a plane, find a fourth point such that the sum of its distances to the three given points is as small as possible'.
Torricelli was the first person to create a sustained vacuum and to discover the principle of a barometer. In 1643 he proposed an experiment, later performed by his colleague Vincenzo Viviani, that demonstrated that atmospheric pressure determines the height to which a fluid will rise in a tube inverted over the same liquid. This concept led to the development of the barometer. Torricelli wrote a letter to his friend Michelangelo Ricci, who like him had been a student of Castelli, on 11 June 1644. At this stage Torricelli was in Florence, writing to his friend Ricci who was in Rome.
I have already called attention to certain philosophical experiments that are in progress ... relating to vacuum, designed not just to make a vacuum but to make an instrument which will exhibit changes in the atmosphere, which is sometimes heavier and denser and at other times lighter and thinner. Many have argued that a vacuum does not exist, others claim it exists only with difficulty in spite of the repugnance of nature; I know of no one who claims it easily exists without any resistance from nature.
Whether a vacuum existed was a question which had been argued over for centuries. Aristotle had simply claimed that a vacuum was a logical contradiction, but difficulties with this had led Renaissance scientists to modify this to the claim that 'nature abhors a vacuum' which is in line with those who Torricelli suggests believe a vacuum exists despite 'the repugnance of nature'. Galileo had observed the experimental evidence that a suction pump could only raise water by about nine metres but had given an incorrect explanation based on the "force created by a vacuum". Torricelli then described an experiment and gives for the first time the correct explanation:-
We have made many glass vessels ... with tubes two cubits long. These were filled with mercury, the open end was closed with the finger, and the tubes were then inverted in a vessel where there was mercury. .. We saw that an empty space was formed and that nothing happened in the vessel where this space was formed ... I claim that the force which keeps the mercury from falling is external and that the force comes from outside the tube. On the surface of the mercury which is in the bowl rests the weight of a column of fifty miles of air. Is it a surprise that into the vessel, in which the mercury has no inclination and no repugnance, not even the slightest, to being there, it should enter and should rise in a column high enough to make equilibrium with the weight of the external air which forces it up?
He attempted to examine the vacuum which he was able to create and test whether sound travelled in a vacuum. He also tried to see if insects could live in the vacuum. However he seems not to have succeeded with these experiments.
In De motu gravium Ⓣ which was published as part of Torricelli's 1644 Opera geometrica Ⓣ, Torricelli also proved that the flow of liquid through an opening is proportional to the square root of the height of the liquid, a result now known as Torricelli's theorem. It was another remarkable contribution which has led to some suggesting that this result makes him the founder of hydrodynamics. Also in De motu gravium Torricelli studied projectile motion. He developed Galileo's ideas on the parabolic trajectory of projectiles launched horizontally, giving a theory for projectiles launched at any angle. He also gave numerical tables which would help gunners find the correct elevation of their guns to give the required range. Three years later he received a letter from Renieri of Genoa who claimed that he had conducted some experiments which contradicted the theory of parabolic trajectories. The two corresponded on the topic with Torricelli saying that his theory was in fact based on ignoring certain effects which would make the experimental data slightly different.
Torricelli not only had great skills in theoretical work but he also had great skill as a maker of instruments. He was a skilled lens grinder, making excellent telescopes and small, short focus, simple microscopes, and he seems to have learnt these techniques during the time he lived with Galileo. Gliozzi writes in [1]:-
... one of Torricelli's telescope lenses ... was examined in 1924 ... using a diffraction grating. It was found to be of exquisite workmanship, sos much so that one face was seen to have been machined better than the mirror taken a reference surface ...
In fact he made much money from his skill in lens grinding in the last period of his life in Florence and the Grand Duke gave him many gifts in return for scientific instruments.
Much of Torricelli's mathematical and scientific work has not survived, mainly because he published only the one work we referred to above. In addition to letters which have survived which tell us important facts about his achievements, we also have some lectures which he gave. These were collected and published after his death and include one he gave when he was elected to the Accademia della Crusca in 1642 and seven others given to the Academy during the next few years. One of these was on the wind and it is important for again Torricelli was the first to give the correct scientific explanation when he proposed that
... winds are produced by differences of air temperature, and hence density, between two regions of the earth.
We referred above to the argument between Torricelli and Roberval concerning the cycloid, and in 1646 Torricelli began gathering together the correspondence which had passed between the two on the topic. It is clear that Torricelli was an honest man who felt that he needed to publish the material to present the truth to the world. There can be no doubt that these two great mathematicians had made similar discoveries about the cycloid but neither had been influenced by the other's ideas. However, before he completed the task of preparing the correspondence for publication Torricelli contracted typhoid in October 1647 died a few days later at the young age of 39 while in his prime as a research mathematician and scientist.
Hours before his death he tried to ensure that his unpublished manuscripts and letters be given to someone to prepare for publication and he entrusted them to his friend Ludovico Serenai. After neither Castelli nor Michelangelo Ricci would undertake the task and although Viviani did agree to prepare the material for publication he failed to accomplish the task. Some of Torricelli's manuscripts were lost and it was not until 1919 that the remaining material was published as Torricelli had wished. His collected works were published with Gino Loria and Guiseppe Vassura as editors, three volumes being published in 1919 and the fourth volume in 1944 nearly 300 years after Torricelli's death. Sadly material left by him, bearing his own signature, was destroyed in the Torricelli Museum in Faenza in 1944.
Torricelli's remarkable contributions mean that had he lived he would certainly have made other outstanding mathematical discoveries. Collections of paradoxes which arose through inappropriate use of the new calculus were found in his manuscripts and show the depth of his understanding. In fact he may indeed have made contributions which will never be known, for the full range of his ideas were never properly recorded.
Please ask all questions before you bid. Most of my items are Estate Finds, they are sold AS-IS. What you see is what you get, if it is not shown and not mentioned it is most likely not included; ask if in doubt. Items listed are already packed and I can therefore not combine shipping unless it is stated in the listing.
Package weighs 8 lbs and 0 ounces and measures 38 by 12 by 12 inches.
To calculate USPS postage to your destination,
click here!
If you find a cheaper way, send me an email indicating the service you want and the price you figured and I will send you a new invoice. However, eBay requires me to post size and weight as I list an item; items are therefore already packed and ready to be shipped. I can therefore not combine shipping, sorry!
PayPal is the default payment method, but
OTHER METHODS ACCEPTED! Please no e-checks or credit card payments through PayPal;
checks only accepted through the mail. There is no handling charge. Shipping costs is depending on destination zip code. I have never had any problems with breakage and pack very carefully. On principal,
I WILL NOT ISSUE A PARTIAL REFUND
for any reason; just mail it back, no questions asked, and you will get a full refund of the purchase price. Thanks for looking...
(refno 0957)